

Holding Period Return (HPR) is a measure of the total return on an investment over a specific period of time, regardless of whether the investment is held for a short or long duration. It takes into account both income earned from the investment (such as dividends or interest) and capital gains or losses due to price changes during the holding period.
HPR is particularly useful for assessing the performance of an investment over a discrete time period, like a year, month, or any other specified time frame. It’s often used in investment analysis to compare the performance of different assets, portfolios, or investment strategies.
The basic formula for calculating the Holding Period Return (HPR) is:
Where:
Example 1: Stock Investment with Dividends
Suppose an investor buys 100 shares of a stock for $50 per share at the beginning of the year. During the year, the stock pays $2 per share in dividends, and by the end of the year, the stock price rises to $60 per share.
Now, plug the values into the HPR formula:
In this case, the Holding Period Return is 24%, meaning the investor achieved a 24% return on the investment over the year, considering both capital appreciation and dividends.
Example 2: Bond Investment with Interest
Let’s assume an investor purchases a bond for $1,000. Over the next year, the bond pays $50 in interest (coupon payment), and the price of the bond rises to $1,050.
Now, apply the HPR formula:
In this case, the Holding Period Return is 10%.
The Holding Period Return is widely used for several reasons:
When an investment is held for less than or more than a year, it is common to annualize the holding period return to make it comparable to annualized returns from other investments. The annualization process adjusts the return to reflect a full year, assuming the investment’s performance over the holding period would continue at the same rate.
To annualize a return, you can use the following formula:
Where:
Example: Annualizing a Six-Month Return
Let’s say an investor has a holding period return of 10% for an investment held for 6 months. To annualize the return, use the formula:
In this example, the annualized holding period return is 21%, assuming the same performance would continue for the full year.
While HPR is a useful measure, it has some limitations:
HPR is often used in the following scenarios:
The Holding Period Return (HPR) is an essential metric for measuring the total return of an investment over a specific period. By including both income and capital gains or losses, HPR provides a comprehensive picture of an investment’s performance. While HPR is a simple and effective tool for performance assessment, investors should be aware of its limitations, including its lack of consideration for compounding, risk, and transaction costs. Annualizing the return can make it more comparable to other investments held over different periods. HPR remains a fundamental calculation for comparing the performance of various assets and for evaluating the success of investment strategies.
$$\begin{aligned} HPR\; &= \left [ Final\;Price\;-\;Original\;Price\;+\;Income \over Original\;Price \right ] \\\\ &= \;\left [ Capital\;Gain\;+\;Dividends \over Original\;Price \right ] \end{aligned}$$
As dividends are paid after a company has paid it’s company tax, the dividend may contain a franking (imputation) credit.
If the dividend is fully franked (100%) investors are entitled to receive the full credit of the tax paid on the dividend as franking (imputation) credits.
Depending on an investors’ individual circumstances, franking credits may be used to decrease the income tax payable by the investor or potentially be received by the investor as a tax refund.
$$ Franking\;Credit = Franked\;Dividend \times \left (Company\;Tax\;Rate \over 1-Company\;Tax\;Rate \right ) $$

See also: Dividends

There are primarily two types of orders placed on the market.
A payment paid regularly by a company to its shareholders out of its profits (or reserves). Interim dividends are generally paid out of surplus profits (reserved) of the previous years, whereas final dividends are declared and paid out on an annual basis after the earnings are known for that financial year. Additionally, companies may pay a bonus dividend.
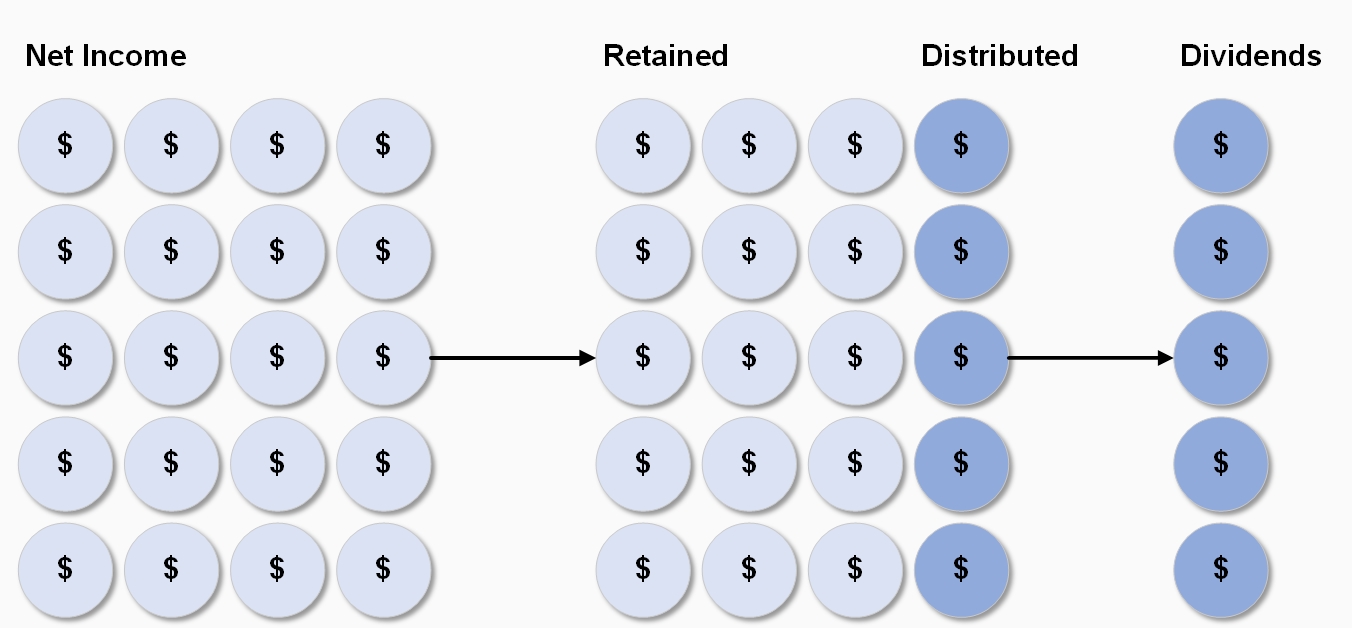
A company typically divides its profits between itself and its shareholders. Distributions represent a portion of the profits a company decides to give to its shareholders, while retained earnings represent the portion of profits that a company chooses to keep. Companies choose to share profits in the form of dividends because it encourages shareholders to continue investing in the company. Understanding the transactions pertaining to dividends and retained earnings helps you know the effects of the transactions on a company’s financial statements.
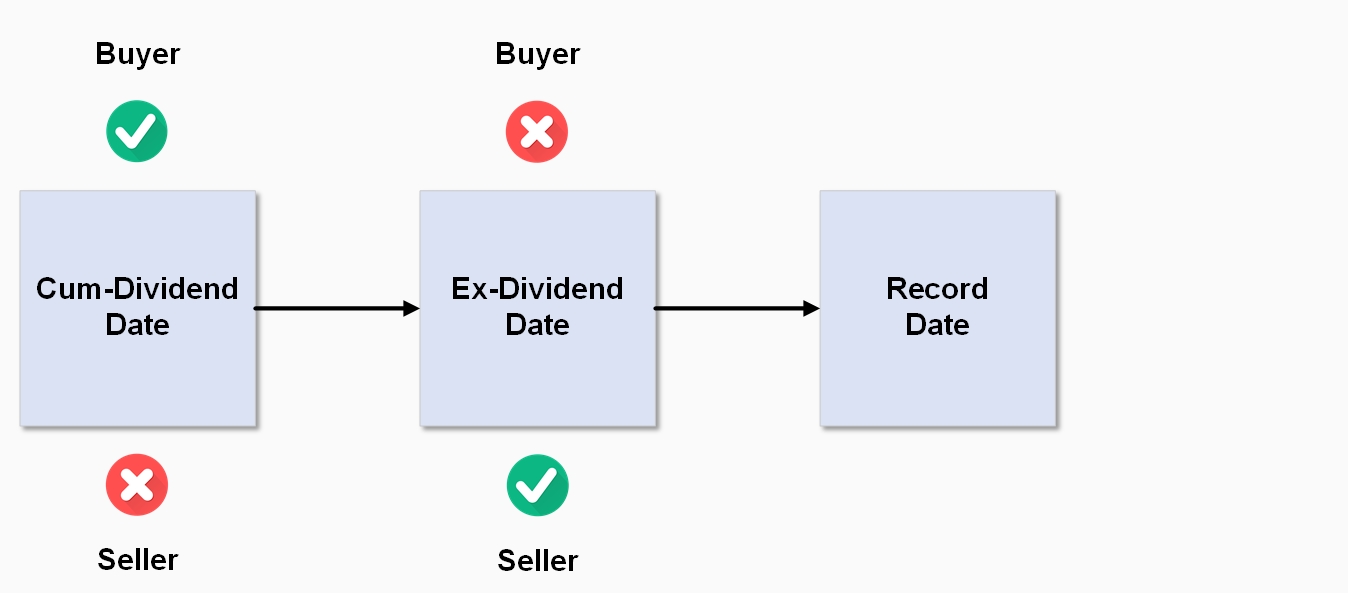
The ex-dividend date occurs one business day before the company’s Record Date.
Important: To be entitled to the dividend, the buyer needs to purchase the shares prior to the ex-dividend date! If you purchase shares on the ex-dividend date, the seller will be entitled to the dividend payment.
The record date is 5.00pm on the date a company closes its share register to determine which shareholders are entitled to receive the current dividend. It is the date where all changes to registration details must be finalised.
$$\begin{aligned} Dividend\;Yield\; &=\;\left [ (Interim\;Dividend + Final\;Dividend) \over Current\;Share\;Price\right ] \;*\;100\;\\\\\ &= \;\left [ Dividends \over Current\;Share\;Price \right ] \;*\;100\;\end{aligned}$$
See also: Franking (Imputation) Credits
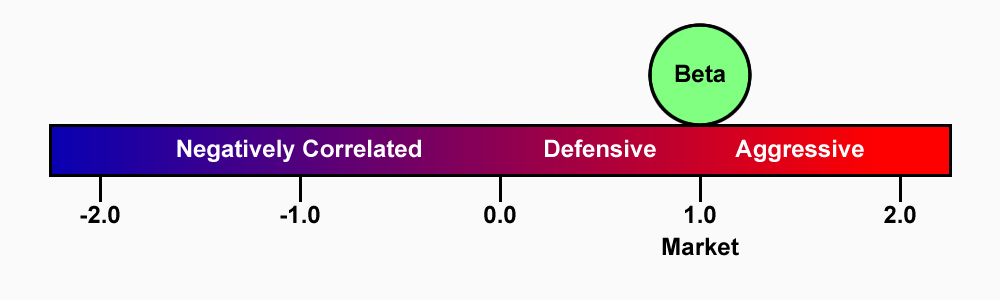
The Beta Coefficient measures the volatility of a particular share (systematic risk) in comparison to the market (unsystematic risk). It describes the sensitivity of a security’s returns in response to swings in the market.
Systematic risk is the underlying risk that affects the entire market. Large changes in macroeconomic variables, such as interest rates, inflation, GDP, or foreign exchange, are changes that impact the broader market and that cannot be avoided through diversification. The Beta coefficient relates ‘the market’ systematic risk to ‘stock-specific’ unsystematic risk by comparing the rate of change between ‘the market’ and ‘stock-specific’ returns.
Statistically, beta represents the slope of the line through a regression of data points from an individual stock’s returns against those of the market.
The beta calculation is used to help investors understand whether a stock moves in the same direction as the rest of the market, and how volatile or risky it is compared to the market.
For beta to provide any insight, the ‘market’ used as a benchmark should be related to the stock.
For example, calculating a bond ETF’s beta by using the S&P 500 as the benchmark isn’t helpful because bonds and stocks are too dissimilar. The benchmark or market return used in the calculation needs to be related to the stock because an investor is trying to gauge how much risk a stock is adding to a portfolio.
A stock that deviates very little from the market doesn’t add a lot of risk to a portfolio, but it also doesn’t increase the theoretical potential for greater returns.
The beta of the market portfolio is always 1.0
$$ Beta\;Coefficient\;(β) = \left [Covariance (rp, rb) \over Variance (rb) \right ]$$